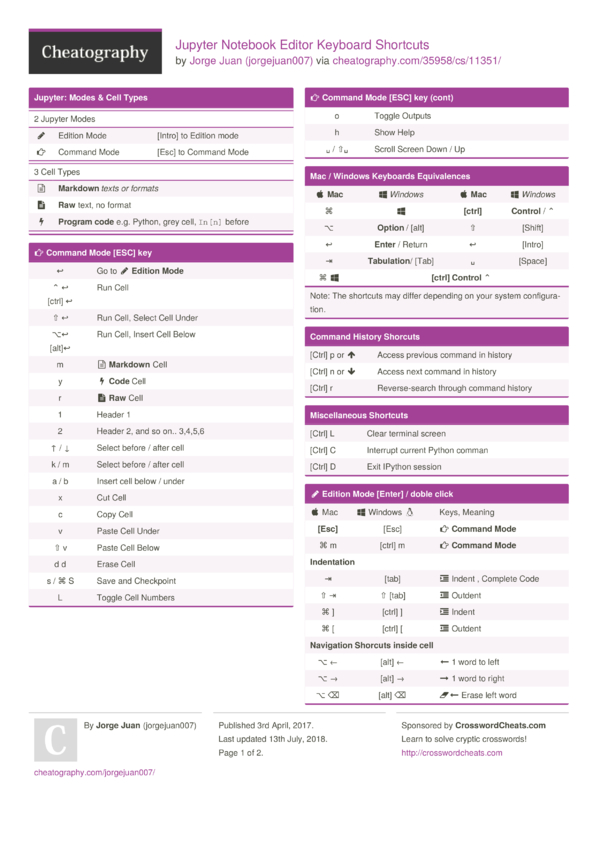
- Jupyter Cheat Sheet Pdf
- Jupiter Notebook Cheat Sheet
- Jupyter Notebook Cheat Sheet
- Jupyter Notebook Cheat Sheet Github
Python For Data Science Cheat Sheet Widgets Jupyter Notebook Learn More Python for Data Science Interactively at www.DataCamp.com Kernels provide computation and communication with front-end interfaces like the notebooks. There are three main kernels: Installing Jupyter Notebook will automatically install the IPython kernel. Create new notebook. Jupyter Notebook files Notebooks written entirely in Markdown Custom notebook formats and Jupytext reStructuredText files Build your book Build your book Execute your computational content Formatting code outputs. # MyST Cheat Sheet. Level 1-6 headings, denoted by number of #. Jupyter Notebook Cheat Sheet This Jupyter Notebook cheat sheet will help you to find your way around the well-known Notebook App, a subproject of Project Jupyter. You'll probably know the Jupyter notebooks pretty well - it's one of the most well-known parts of the Jupyter ecosystem!
Jupyter Notebook is a great tool for data analysis. And one of its greatest features is that you can easily combine runnable code with rich-text annotations. Markdown cells that contain such annotations support both HTML and Markdown syntax.
Personally I prefer # Header (markdown syntax) to <h1>Header</h1> (HTML syntax) in my notebook’s cells as the former looks more human. So I use markdown syntax more often and a concise cheat sheet for Jupyter Notebook markdown is what I was missing for a long time. Yes, I’m aware of such comprehensive cheat sheets like this and this, they are great but too verbal and it’s hard to print them on one page to have it easily accessible.
Eventually, I decided to gather all crucial points of the markdown syntax that are actual for Jupyter notebook and combine them in a concise cheat sheet that I present to you. It doesn’t explain much but should be useful if you simply forgot something. Click the picture to download the PDF version.
I didn’t get into the details of LaTeX syntax (see the penultimate cell in the cheat sheet with the square root of k) since it’s well described here (get ready, it’s a very long document) and you can even download a pretty good cheat sheet here.
Jupyter Cheat Sheet Pdf
This document will be available to you during tests and exams
7.1. Table of Contents¶
7.2. Numeric¶
7.3. Basic plotting functions¶
7.4. Symbolic manipulation¶
Jupiter Notebook Cheat Sheet
7.4.1. Imports¶
Symbol definitions
Example controller and system
7.4.2. Working with rational functions and polynomials¶
We often want nice rational functions, but sympy doesn’t make expressions rational by default
The cancel function forces this to be a fraction. collect collects terms.
In some cases we can factor equations:
Obtain the numerator and denominator:
If you want them both, you can use
Convert to polynomial in s
Once we have a polynomial, it is easy to obtain coefficients:
Calculate the Routh Array
To get a function which can be used numerically, use lambdify:
7.4.3. Functions useful for discrete systems¶
Write in terms of positive powers of (z):
Write in terms of negative powers of (z):
Inversion of the (z) transform
7.5. Equation solving¶
7.5.1. Symbolic¶
7.5.2. Numeric sympy¶
7.5.3. Numeric¶
7.6. Matrix math¶
7.6.1. Symbolic¶
Creation
Determinant, inverse, transpose
Math operations: Multiplication, addition, elementwise multiplication:
7.6.2. Numeric¶
Creation
Jupyter Notebook Cheat Sheet
Determinant, inverse, transpose
Jupyter Notebook Cheat Sheet Github
Math operations: Multiplication, addition, elementwise multiplication:




